Muon transfer

Due to the differences in the binding energies, the muon can be transferred to the heavier isotopes:

$$(\mu x)_{nl} + y \rightarrow x + (\mu y)_{nl'} + \frac{\Delta E_{xy}}{n^{2}},$$ (2)

where $\Delta E_{xy}$ is the isotope splitting (Table 1.1) due to the reduced mass difference. The subscript n denotes the possibility of transfer from excited states (Section 1.2.4). In the D/T mixture target, the rate of muon transfer from the ground state of the deuterium atom to the triton $\lambda _{dt}$ is relatively slow ( $\sim 3\times 10^{8}$ s^-1) and becomes one of the bottle-necks at low tritium concentrations. It should be noted that the released energy, divided between two projectiles, gives an acceleration to the muonic atom in the lab frame corresponding to the energy

$$E(\mu y)^{Lab} = \frac {m_{x}} {m_{x}+m_{\mu y}} \frac {\Delta E_{xy}}{n^{2}}.$$ (3)

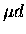The energy dependent calculations predict that $\lambda _{dt}$ is significantly larger at higher energies (more than about 10 eV). This may be exploited, for example, by triple isotope mixture targets (H/D/T) [27], in which a faster reaction, muon transfer from a proton to a deuteron, creates an energetic $\mu d$, thus effectively speeding up the transfer to tritium.

  

Figure 1.2: Scattering cross sections for $\mu t$ with a hydrogen isotope nuclei from the Nuclear Atlas [16,17], showing the Ramsauer-Townsend minimum at around 10 eV for $\mu t + p$. $\mu t (F) + t$ cross sections plotted include both elastic and spin exchange reactions, where $\mu t$(0) is the singlet state and $\mu t$(1) is the triplet state.