Hylleraas type basis expansion

A popular choice are Hylleraas type functions [106,107,108,109], which for J=0 states read

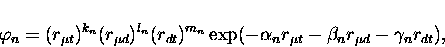$$\varphi _{n} = (r_{\mu t})^{k_{n}} (r_{\mu d})^{l_{n}} (r_{... ...ha _{n} r_{\mu t} -\beta _{n} r_{\mu d} -\gamma _{n} r_{d t}),$$ (34)

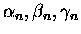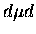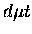and are expressed in the interparticle co-ordinates, where r_xy denotes the distance between the particle x and y. The exponential parameters $\alpha _{n} , \beta _{n}, \gamma _{n}$ are often taken to be the same for all n to reduce the number of parameters to be optimized. For loosely bound states (1,1) of $d\mu d$ and $d\mu t$, similar functions with k_i=l_i=m_i, known as Slater gemials were found to be more useful in representing the large physical size of the states [110,84]. The disadvantage with Slater gemials is that one has to optimize a large number of exponential parameters, which is very time consuming. Another disadvantage is its ``linear dependencies'' problem. This is due to the fact that the basis in this set is nearly linearly dependent, i.e. rather non-orthogonal. Since the functions differ only by their exponents, an optimized basis set sometimes has several functions with close values of the exponents [85]. Thus, the use of extended arithmetic precision ($\sim 30$ decimal digits) is necessary.