UNIVERSITY OF BRITISH COLUMBIA

Science 1 Physics

Third Midterm - SOLUTIONS

29 February 2000

Time: 50 minutes

Instructors: Jess H. Brewer & Domingo Louis-Martinez

1.
Plane Wave   [20 marks] A plane electromagnetic wave is traveling in the positive x direction at speed $c = 2.997 \times 10^8$ m/s. If we take a ``snapshot'' of the wave at t=0, we find that the electric field E is +0.7071 volts per meter (V/m) at x=0 and increases in the region from x=0 to x=1 m, where it reaches its maximum value of +1.0 V/m. Write down a formula for the electric field E(x,t) as a function of position x and time t, giving quantitative values for each of the symbols in the formula. (A sketch may be helpful.)

ANSWER:


 
Figure: - ``Snapshot'' at t=0.
\begin{figure}\begin{center}\epsfysize 2in \mbox{\epsfbox{PS/pi_4-phase.ps}} \end{center} \end{figure}

The title of the question is all you need to write down the formal solution:
\fbox{ $ E(x,t) = E_\circ \cos(kx - \omega t + \phi) $ . }
(You can use the imaginary exponential form if you like: $ E(x,t) = E_\circ e^{i(kx - \omega t + \phi)} $.)

The amplitude $E_\circ$ is the maximum value of E: \fbox{ $E_\circ = 1$ ~V/m. }

At t=0 and x=0 we have $0.7071 = E_\circ \cos \phi$ so $\cos \phi = 1/\sqrt{2}$ so $\phi = \pm \pi/4$ ($45^\circ$ for Philistines). Which sign? Well, adding a positive kx makes $\cos (kx + \phi)$ bigger, so the initial phase must be negative: \fbox{ $\phi = - \pi/4$ . }

Since $kx = \pi/4$ when x=1 m, we have \fbox{ $k = \pi/4 \hbox{\rm ~m}^{-1}$ . } (This can also be ``read off the graph'' since $k \equiv 2\pi/\lambda$ and $\lambda = 8$ m by inspection.)

Finally, $\omega = ck = 2.997 \times 10^8 \times \pi/4$ or \fbox{ $\omega = 2.355 \times 10^8 \hbox{\rm ~s}^{-1}$ . }

2.
Particle in a Box   [20 marks] In 1924, Louis Victor Pierre Raymond duc de Broglie suggested that if light behaves like particles then perhaps electrons behave like waves, with a universal relationship between momentum p and wavelength $\lambda$ given by   $p \lambda = h$  (where h is Planck's constant).
(a)
[10 marks]   An electron is confined to a one-dimensional box of length L = 1 nm. Taking de Broglie's hypothesis into account, what is the lowest kinetic energy it can have? (Express your answer in electron volts.)

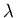ANSWER: The kinetic energy of a nonrelativistic electron is given in terms of its momentum and mass by E = p2/2m. (We can provisionally assume it is nonrelativistic and check our result later to make sure that E is much less than me c2 = 511 keV.) Thus we get the smallest E for the smallest p. In turn, we get the smallest p for the largest $\lambda$, and the largest lambda we can ``fit into the box'' with nodes at both ends is $\lambda_{\rm min} = 2L$. 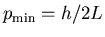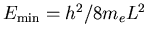Thus $p_{\rm min} = h/2L$ and so $E_{\rm min} = h^2/8m_e L^2$. 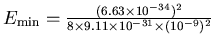Plugging in the numbers (1 nm = 10-9 m) gives $E_{\rm min} = {(6.63 \times 10^{-34})^2 \over 8 \times 9.11 \times 10^{-31} \times (10^{-9})^2 }$ = $0.6022 \times 10^{-19}$ J. 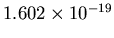Since 1 eV = $1.602 \times 10^{-19}$ J, \fbox{ $E_{\rm min} = 0.376$ ~eV. }

(b)
[6 marks]   What happens to the electron's energy if L shrinks by a factor of ten?

ANSWER: The minimum momentum grows by a factor of ten and the minimum energy grows by a factor of 100: 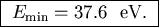\fbox{ $E_{\rm min} = 37.6$ ~eV. } (Compare the ionization potential of the hydrogen atom, 13.6 eV.) This is still tiny compared to the electron's rest mass energy of 511 keV, so our assumption of nonrelativistic kinematics was OK.

(c)
[4 marks]   What sort of objects are about this size?

ANSWER: Atoms! A box 0.1 nm across is roughly the same size as Bohr's H atom (0.1 nm = 10-10 m = 1 Å). The ionization potential of the H atom is smaller than the $E_{\rm min}$ we calculated ``sort of because'' there is more ``room'' in 3D than in 1D.

3.
Concentric Spherical Shells   [30 marks]

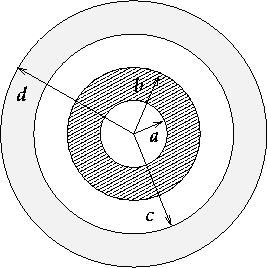
A hollow conducting sphere has an inner radius  a  and an outer radius  b.  It is enclosed within a concentric hollow sphere of inner radius  c  and outer radius  d,   made of an insulating material with dielectric constant  $\kappa$.

A charge  Q  is placed on the conductor.

Express the electric field   $\vec{\hbox{\boldmath$E$\unboldmath }}$  as a function of radius  r  (and other constants) in each of the five regions:  r<a,   a<r<b,   b<r<c,   c<r<d  and  r>d.

\begin{figure}\begin{center}\epsfysize 2.333in \mbox{\epsfbox{PS/2shells.eps}} \end{center}\end{figure}

ANSWER: This problem can be solved ``by inspection'' but a little explanation is always a good idea. (There can be no part credit without it.) As usual, I'll give a far more verbose explanation than you should.

First, by spherical (isotropic) symmetry, the vector electric field is radial everywhere. (If you wanted to pick a favoured direction, how would you choose it?) Mathematically, \fbox{ $\vec{\hbox{\boldmath$E$\unboldmath}} = E \hat{r}$ . } All that remains is to find the scalar magnitude of the field, E(r).

Now apply Gauss' Law: 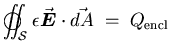${\displaystyle \int\!\!\!\!\int_{\cal S}\!\!\!\!\!\!\!\!\!\bigcirc \; \; \epsilon \vec{\hbox{\boldmath$E$\unboldmath }} \cdot \vec{dA} \; = \; Q_{\rm encl} }$, where $\epsilon = \kappa \epsilon_\circ$ automatically accounts for any dielectric effects. (In vacuum, $\kappa = 1$.)

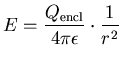For a spherical Gaussian surface ${\cal S}$ at radius r, the left-hand side is just $4 \pi r^2 \epsilon E$, so our general expression of Gauss' Law is ${\displaystyle E = {Q_{\rm encl} \over 4\pi \epsilon} \cdot {1 \over r^2} }$. Now for the 5 regions:

r < a: $Q_{\rm encl} = 0$ so \fbox{ $E=0$ . }

a < r < b: \fbox{ $E=0$\space } inside any conductor (otherwise charges would move until it was). All the Q goes to the outer surface of the conductor - as far away from itself as it can get.

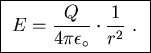
b < r < c: \fbox{ ${\displaystyle E = {Q \over 4\pi \epsilon_\circ} \cdot {1 \over r^2} }$ . }

c < r < d: 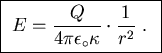\fbox{ ${\displaystyle E = {Q \over 4\pi \epsilon_\circ \kappa} \cdot {1 \over r^2} }$ . } Note that E actually decreases upon entering the dielectric, due to the induced negative surface charge at r=c, then ``recovers'' upon exit, due to the matching positive surface charge at r=d.

r > d: 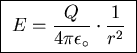\fbox{ ${\displaystyle E = {Q \over 4\pi \epsilon_\circ} \cdot {1 \over r^2} }$\space } just as in region b < r < c.

4.
Velocity Selector   [30 marks]

A beam of protons with velocity v = 106 m/s is directed along the +x axis between a pair of square metal plates of area A = 1 m2 separated by a distance d = 1 cm. The plates have an initial charge of Q = 10-6 C (the left plate has +Q and the right plate has -Q). A uniform magnetic field B = 0.01 T is applied in the +z direction. At t=0 the switch S is closed, allowing the charge to flow off the plates through an R = 100 M$\Omega$ resistor.

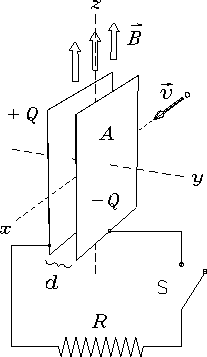
\begin{figure}\begin{center}\epsfysize 3.1in \mbox{\epsfbox{PS/dc_sep-circuit.ps}} \end{center}\end{figure}


Assuming that the magnetic field acts only in the space between the plates, and neglecting ``edge effects'' on the electric field between the plates, calculate the time at which the protons will pass undeflected between the plates.

ANSWER: The beam passes undeflected if v = E/B, so we want E = 104 V/m. What is the initial E0? For parallel plates we have generally $E = \sigma / \epsilon_\circ = Q/\epsilon_\circ A$. Initially Q = 10-6 C, and A = 1 m2, so E0 = (105/0.8854) V/m, larger than needed. This is good, since when we close the switch and bleed off Q, E will decrease exponentially with time constant $\tau = RC$. What is $\tau$? Well, for a parallel plate capacitor the capacitance is given by $C = \epsilon_\circ A/d$ which in this case gives $C = {0.8854 \times 10^{-11} \times 1 \over 10^{-2}} = 0.8854 \times 19^{-9}$ F, and $R = 10^8 \; \; \Omega$, so $\tau = 0.08854$ s. How long does it take for E to drop from E0 to the desired value? $E(t) = E_0 e^{-t/\tau}$ so $10^4/E_0 = 0.08854 = e^{-t/\tau}$. Taking the log of both sides gives $\ln(0.08854) = -2.4243 = -t/0.08854$ or \fbox{ $t = 0.2146$ ~s. }

Note: if you forgot any of the formulae above, they are all ``one-liner'' derivations from definitions and first principles.


Jess H. Brewer
2000-03-03