As an exercise in the "brute force" integration of
COULOMB'S LAW (unavoidable in most cases), here is
one way to find the electric field due to a uniformly charged,
skinny rod of finite length ![]() :
:
If the total charge ![]() is uniformly distributed along the rod,
then the charge per unit length is
is uniformly distributed along the rod,
then the charge per unit length is
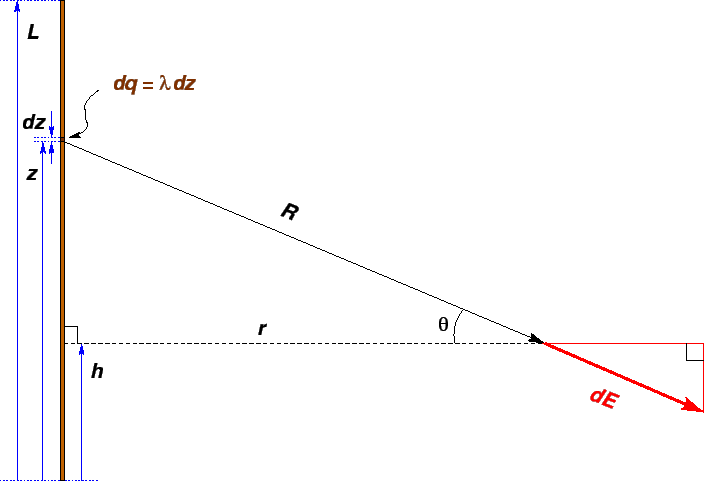
Then we pick an arbitrarily position a distance ![]() up from the bottom
end of the rod, as shown. A small slice of the rod (width
up from the bottom
end of the rod, as shown. A small slice of the rod (width ![]() )
at that position contains a "charge element"
)
at that position contains a "charge element"
![]() which contributes
which contributes ![]() to the electric field vector
to the electric field vector ![]() at the test point. Coulomb's Law says that
at the test point. Coulomb's Law says that ![]() points away
from the charge element (assuming positive charge) and has a magnitude
points away
from the charge element (assuming positive charge) and has a magnitude
If we want to solve this problem without reference to external aids (like tables of integrals), it is better to convert into angles and trigonometric functions as follows:
Equation (6) can be rewritten
and since giving we can write Eq. (2) as and from that, Eqs. (4) and (5), respectively, as where
Integrating these differentials is trivial; we are left with just
the differences between ![]() (or
(or ![]() ) at the limits of integration
(the top and bottom of the rod):
) at the limits of integration
(the top and bottom of the rod):
(note that
These equations express a completely general solution to this problem.
Let's check to see what these give for the field directly out from the
midpoint of the rod - i.e. for ![]() :
:
Let's also check to see what we get for ![]() (at the midpoint)
very far from the rod (
(at the midpoint)
very far from the rod (![]() ):
):
and very close to the rod (![]() ):
):