BELIEVE   ME   NOT!    -     A   SKEPTIC's   GUIDE  

Fudging The Bohr Atom

If the electron travels in a circular path (as postulated by Niels Bohr in 1913) then we must apply de Broglie's hypothesis in a slightly different way: namely, the electron's "wave" must be single valued -- it has to get back to the same value as it travels around the closed loop back to where it started. This means that the circumference of the loop is an integer number of wavelengths, or

$$2 \pi r_n \; = \; n \lambda_n$$ (24.7)

where r_n is the radius of the orbit for the $n^{\rm th}$ allowed mode. This in turn predicts a relationship between the radius and the momentum,

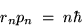$$r_n p_n \; = \; n \hbar$$ (24.8)

where 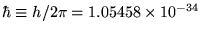$\hbar \equiv h/2\pi = 1.05458 \times 10^{-34}$ J-s. [Actually in any sensible system of units $\hbar = 1$, just like c = 1, but we are forced by tyrannical bureaucrats and twisted social conventions to use SI units.]

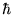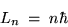But what is the product of the radius and the momentum for a circular orbit? The ANGULAR MOMENTUM! Thus Voila! We have Bohr's hypothesis, namely that angular momentum L is quantized in units of $\hbar$:

$$L_n \; = \; n \hbar$$ (24.9)