BELIEVE   ME   NOT!    -     A   SKEPTIC's   GUIDE  

Periodic Behaviour

Nature shows us many "systems" which return periodically to the same initial state, passing through the same sequence of intermediate states every period. Life is so full of periodic experiences, from night and day to the rise and fall of the tides to the phases of the moon to the annual cycle of the seasons, that we all come well equipped with "common sense" tailored to this paradigm.^13.1 It has even been suggested that the concept of time itself is rooted in the cyclic phenomena of Nature.

In Physics, of course, we insist on narrowing the definition just enough to allow precision. For instance, many phenomena are cyclic without being periodic in the strict sense of the word.^13.2 Here cyclic means that the same general pattern keeps repeating; periodic means that the system passes through the same "phase" at exactly the same time in every cycle and that all the cycles are exactly the same length. Thus if we know all the details of one full cycle of true periodic behaviour, then we know the subsequent state of the system at all times, future and past. Naturally, this is an idealization; but its utility is obvious.

   Figure: Some periodic functions.

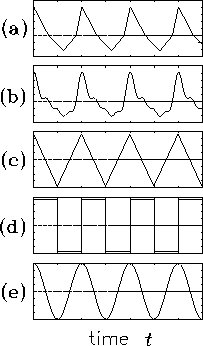
Of course, there is an infinite variety of possible periodic cycles. Assuming that we can reduce the "state" of the system to a single variable "q" and its time derivatives, the graph of  q(t)  can have any shape as long as it repeats after one full period. Fig. 13.1 illustrates a few examples. In (a) and (b) the "displacement" of  q away from its "equilibrium" position [dashed line] is not symmetric, yet the phases repeat every cycle. In (c) and (d) the cycle is symmetric with the same "amplitude" above and below the equilibrium axis, but at certain points the slope of the curve changes "discontinuously." Only in (e) is the cycle everywhere smooth and symmetric.