A solution

In an attempt to resolve this discrepancy, we revisit the relative amplitudes in the telescope measurements. As discussed in Section 6.4.2 (p. ), the telescope data gave some 30% higher value of the reduced stopping fraction , compared to the electron scintillator data. We suspect that this might be due the energy sensitivity of the detectors to the stopping fraction, as the telescope events required a certain energy deposit in the liquid scintillator. This was experimentally demonstrated in Fig. 6.6 (page ), and qualitatively explained to be due in part to the muon decay electron energy spectra (p. ).

Here we postulate the possibility of muon-capture related processes still not fully accounted for by various corrections which we have made in Section 6.4.3. One such example is gamma induced reactions in the target.

 

We make use of the minimum ionizing peak ( MeV) in the telescope energy spectra, and make a comparison with the GEANT simulations of full electronic processes, to extract the relative amplitude. The possible advantages of using the energy cuts at this peak, as opposed to no energy cuts, include: (a) energy deposited from a muon-capture related process, if it exists, should be less important at high energies, and (b) with the lack of an energy calibration for the Tel spectra, the minimum ionizing peak itself can provide a calibration. Nevertheless, in order to account for some ambiguity in the exact cut positions corresponding to the experimental energy cut at the peak (590<E<790 ch for both Tel1 and Tel2; see Fig. 6.6), we applied two different sets of cuts in the Monte Carlo spectra. Shown in Fig. 6.8 are the simulated spectra and applied cuts. With these cuts, we determined the relative efficiencies of electron detection necessary to derive the stopping fraction, as given in Table 6.18. The first parentheses in the tables are the Monte Carlo statistical errors, while the second indicate systematic uncertainties due to the different cuts in the Monte Carlo energy spectra. As can be seen, the resulting relative efficiencies are not very sensitive to the choice of the cuts. Note that the charged particle effect ratio of 1 was used for Tel1 (bold face in the table), as opposed to 1.04 in the scintillator measurement, because of the higher energy cuts applied here. The stopping fractions S^AR_H determined with the telescope thus are given in Table 6.19, together with uncorrected values.

 

Table 6.18: The correction factors $\eta$, $\kappa$ in Eq. 6.15, and assumed quantities for the derivation by Eqs. 6.16, 6.17. Values with `*' are taken from the ratio of the efficiencies calculated by GEANT simulation with the cuts given in Fig 6.8. The first parentheses are the Monte Carlo statistical errors, while the second indicate systematic uncertainties due to the different cuts.

Detector				$\eta$	$\kappa$
Tel1	0.600(9)(9)*	1	1	0.510(8)(8)	1.059(18)(5)*
Tel2	0.578(12)(7)*	1	1	0.491(10)(6)	1.188(25)(4)*

 

Table 6.19: The uncorrected stopping fraction $\overline {S}_H ^{AR}$ and the corrected one S_H^AR using the minimum ionizing peak of the telescope detectors in the relative amplitude method. Uncorrected values with nominal energy cuts (200<E<1200 ch) are also given for comparison.

	Energy cuts (%)	Tel1	Tel2
$\overline {S}_H ^{AR}$	nominal	36.3(4)	32.8(4)
$\overline {S}_H ^{AR}$	min. ion. peak	42.4(8)	39.9(7)
SHAR	min. ion. peak	31.3(14)	29.6(14)

 

The results for S^AR_H from the telescope are in good agreement with the absolute amplitude method, while in disagreement with the relative method with the scintillators. The stopping fractions determined from various methods are summarized in Table 6.20. Also listed is the result of a GEANT based beam stopping simulation performed by Marshall assuming the peak momentum of 27.0 MeV/c with (these values were carefully tuned to match the relative hydrogen stopping fractions for a range of beam momentum). Our results suggest that the relative amplitude method, using electron scintillators, may be unreliable for a stopping fraction determination, even with elaborate corrections applied as in the preceding sections, due perhaps to further capture related background such as nuclear gammas which are not accounted for. In fact, a preliminary study by Art Olin using an evaporation model for particle emission following nuclear excitation from muon capture suggests this may be the case. Excluding the scintillator relative amplitude values, we obtain consistent results on the stopping fraction. The absolute amplitude method with its advantages discussed above, appears to be more reliable, hence I shall use the value for the present work.

 

Table 6.20: Summary of stopping fractions determined via various methods. The absolute amplitude result (bold face) will be used for this work.

Method	Detector	SH (%)	Comment
Relative amplitude	En2	23.4(7)	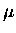$\mu$ capture related background?
Relative amplitude	Tel1, 2	30.5(14)	Energy cut $\sim$16-20 MeV
(min. ionizing peak)
Absolute amplitude	En2, Tel2	29.9(15)	No MC correction necessary
Beam simulation	(GEANT)	31.1	p=27.0 MeV/c, %

 

Finally, I point out that since many of the previous experiments in the field used the simple relative amplitude method, often without any efficiency corrections, their results may require some re-interpretation, particularly when muon stopping in non-hydrogenic materials is significant, as in a low density gas target.