BELIEVE   ME   NOT!    -     A   SKEPTIC's   GUIDE  

Linear Superposition

The above derivation relied heavily on the SMALL-ANGLE APPROXIMATIONS which are valid only for small displacements of the string from its equilibrium position (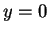$y=0$ for all 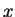$x$). This almost always true: the simple description of a wave given here is only strictly valid in the limit of small displacements from equilibrium; for large displacements we usually pick up "anharmonic" terms corresponding to nonlinear restoring forces. But as long as the restoring force stays linear we have an important consequence: several different waves can propagate independently through the same medium. (E.g. down the same string.) The displacement at any given time and place is just the linear sum of the displacements due to each of the simultaneously propagating waves. This is known as the PRINCIPLE OF LINEAR SUPERPOSITION, and it is essential to our understanding of wave phenomena.

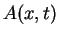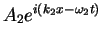In general the overall displacement  $A(x,t)$  resulting from the linear superposition of two waves   $A_1 e^{i(k_1 x - \omega_1 t)}$  and   $A_2 e^{i(k_2 x - \omega_2 t)}$  is given by

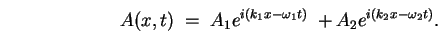$$A(x,t) \; = \; A_1 e^{i(k_1 x - \omega_1 t)} \; + A_2 e^{i(k_2 x - \omega_2 t)} .$$ (14.17)

Let's look at a few simple examples.